Part 1: What to Measure?

In class we said that if you know the radiance along every line segment through free space, you know everything about light in a scene. But sometimes radiance gives you more information than you really need. What is the minimal amount of information needed in each of the following scenarios? For each answer you must write:
- the name of a radiometric quantity (e.g., "radiant energy", "radiant flux", etc.)
- what this radiometric quantity means (e.g., "radiant energy per unit time")
- WHY this quantity is appropriate for the given situation
- Suppose you're going to the beach and want to calculate exactly how much sunscreen to put on each spot on your body in order to avoid a sunburn. What radiometric quantity will help you make this calculation? You can assume you're just going to lay flat on the beach for three hours and not move a muscle (sounds nice!).
- Suppose you have a solar panel on the roof of your house, and you've collected enough sunshine this month that your utility company is paying you to pump it back into the electricity grid. What radiometric quantity do you need to figure out how much they owe you?
- Suppose you want to recreate the most beautiful sunset you've ever seen. What radiometric quantity do you need to capture to accurately reproduce it in a VR headset?
- Suppose you are viewing a distant star through a telescope. What radiometric quantity can you record to learn as much as possible about this star (e.g., its age or distance from Earth)?
Part 2: How Much Light?
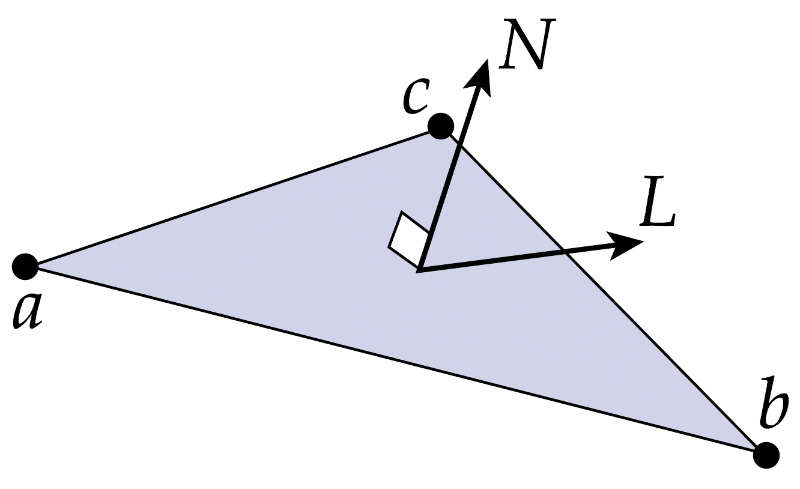
Suppose we have a triangle lit by a directional light source; you want to figure exactly how bright the triangle is. In particular, consider a directional light source with direction
L = (1,0,0)
and intensity
I = 5 W/m2,
and a triangle with vertices
a = (1cm,4cm,7cm),
b = (8cm,2cm,5cm),
c = (6cm,9cm,3cm).
You should compute
- the total radiant flux of the illumination incident on the triangle, as well as
- the irradiance at any point.
[Hint: check your units to make sure you got the right answer! :-)]